Free Energy and Equilibrium Constant Study Guide

Introduction

Gibbs’s free energy and the equilibrium constant are integral principles in the field of thermodynamics. Before we talk about the relationship between Gibbs’s free energy and the equilibrium constant and how to calculate their values, let’s quickly get some background on thermodynamics for a fuller picture of how they work through the concepts of entropy and enthalpy.

Thermodynamics

Thermodynamics studies the relationships between heat, work, temperature, and energy. It describes how the energy of a system changes and whether or not that energy can be used for useful work.

A system is defined as a body of matter or radiation enclosed by permeable walls that separate it from its surroundings, for example, an air conditioner that circulates refrigerant internally and promotes the transfer of heat between itself and its surroundings.

“Work” in the context of thermodynamics is the transfer of energy from a system to its surroundings, as exemplified by the air conditioner system. “Useful” work refers to an exchange of energy that does not involve the expansion of a closed system, meaning that its volume remains the same.

The concepts of entropy and enthalpy are also important principles to understand when learning about Gibbs free energy and the equilibrium constant. Entropy is defined as the thermal energy of a system per unit of temperature which is unavailable for useful work, whereas enthalpy is defined as the total heat of the system.

Now that we’ve got that down, we’re ready to dive into our topic of the day, Gibbs free energy and the equilibrium constant!

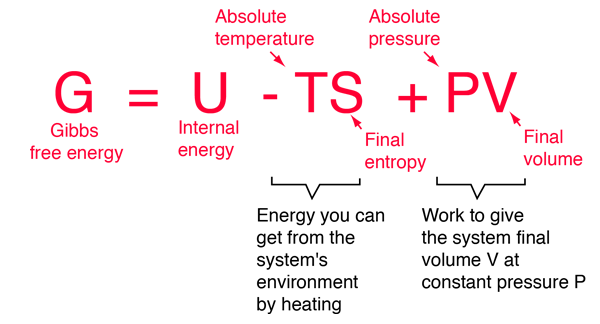
GIBBS FREE ENERGY

• Gibbs free energy, or free energy, is the maximum amount of energy a substance can contribute to a chemical transformation or reaction.
• It is equal to the sum of entropy and the product of temperature in a closed system.
• In simpler words, it is the sum of entropy and enthalpy.
• The change in energy, ΔG, can indicate the direction of chemical reaction under two conditions: constant pressure and constant temperature.
• It can be written as:

G = H−TS

Or more completely as:

G = U + PV−TS

In both cases,

U is internal energy (SI unit: joule)P is pressure (SI unit: pascal)V is the volume (SI unit: m3)T is the temperature (SI unit: kelvin)S is entropy (SI unit: joule/kelvin)H is the enthalpy (SI unit: joule)

Source

EQUILIBRIUM CONSTANT

• The equilibrium constant is the ratio of the concentration of products to the concentration of reactants.
• It is denoted by K(eq).
• The formula of the equilibrium constant is:

K(eq) = Kf / Kb = (C)c (D)d/(A)a (B)b = K(c)

Where K(c) indicates the equilibrium constant measured in moles per liter

RELATION BETWEEN GIBBS FREE ENERGY AND EQUILIBRIUM CONSTANT

When equilibrium is reached, there is no additional free energy change, i.e., ΔG = 0, and Q is equal to the equilibrium constant (K(eq)). Hence the relationship between K(eq) and ΔG becomes:

ΔGo = –RT In K(eq) or ΔGo = –2.303 RT log K(eq)

• Where R is the ideal gas constant (8.314 J/K mol),
• T is the Kelvin temperature,
• And K(eq) is the natural logarithm of the equilibrium constant.

FAQ’s

1. What is the relationship between free energy and equilibrium constant?

When equilibrium is reached, there is no additional free energy change, i.e., ΔG = 0, and Q is equal to the equilibrium constant (K(eq)). Hence the relationship between K(eq) and ΔG becomes:

ΔGo = –RT In K(eq), orΔGo = –2.303 RT log K(eq)

2. How do you calculate free energy?

Calculation of Free energy:ΔG = ΔH − TΔS

3. What is the concept of free energy?

Free Energy refers to the energy in a system that is free to do work, i.e., the internal energy minus any energy that is inaccessible to perform work.

We hope you enjoyed studying this lesson and learned something cool about Free Energy and Equilibrium Constant! Join our Discord community to get any questions you may have answered and to engage with other students just like you! Don’t forget to download our app to experience our fun VR classrooms – we promise it makes studying much more fun!😎

SOURCE

1. Gibbs Free Energy: https://chem.libretexts.org/Bookshelves/Physical_and_Theoretical_Chemistry_Textbook_Maps/Supplemental_Modules_(Physical_and_Theoretical_Chemistry)/Thermodynamics/Energies_and_Potentials/Free_Energy/Gibbs_(Free)_Energy. Accessed 28 Feb 2022

2. Calculations of Free Energy: https://flexbooks.ck12.org/cbook/ck-12-chemistry-flexbook-2.0/section/20.8/primary/lesson/calculations-of-free-energy-and-keq-chem/. Accessed 28 Feb 2022.

]]>