Single Slit Diffraction Study Guide

INTRODUCTION

When we examine light waves, we learn about their many features like, how they reflect back in the process of reflection, how they change direction in the process of refraction, and how they interfere with other light waves. But, what do you believe happens when light waves collide with a gap? Will it go straight through the gap, or will it shift its direction? Let’s investigate.

What is Diffraction?

The process of bending light around corners such that it spreads out and illuminates regions where a shadow is anticipated is known as diffraction of light. In general, because both occur concurrently, it is difficult to distinguish between diffraction and interference. The diffraction of light is what causes the silver line we see in the sky. A silver line appears in the sky as the sunlight penetrates or strikes the cloud.

What Is Single Slit Diffraction?

• The curving of light waves around a tight turn of an obstacle or an opening is known as the diffraction of light.
• The single slit diffraction’s meaning is that an alternating dark and bright pattern can be seen when light is imposed on a slit with a size corresponding to the wavelength of light.
• When light strikes the gap, secondary wavelets form at each point, as per Huygens’ rule.
• These wavelets start out in a phased manner and then disperse on all sides.
• Each one of them covers a specific path to reach any location on the screen.
• Due to the path difference, they reach diverse phases and may interact either constructively or destructively.

SINGLE SLIT DIFFRACTION EXPERIMENT

• The single-slit diffraction experiment is similar to the double-slit performed by Thomas Young in 1801.
• Here, monochromatic light is projected through a slit of finite length.
• The only differences between a single slit and a double-slit experiment are the diffraction patterns and the intensity graphs.

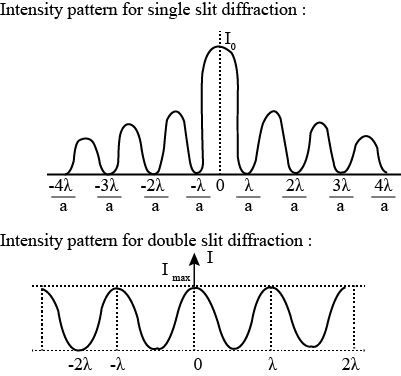
The double-slit experiment patterns differ from the single-slit diffraction pattern as the width and intensity reduce as we move away from the central maximum. 

Single Slit Diffraction Formula

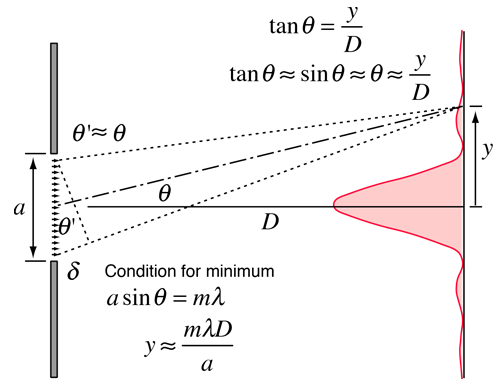
The path difference between two rays at an angle θ is given as ∆L= a/2 sin θ (valid only when D>> a ) 

The bright fringes appear at θ → sin ^-1 ( ± 3ℷ/2), sin^-1 (± 5ℷ/2) 

The dark fringes appear at a sin θ = mℷ

The angular width of the central maximum is 2θ = 2λ/a

The linear width of central maxima is  2λDa.

Here,

∆L is the path difference 

 λ is the wavelength of the projected light 

D is the distance between a slit and a screen 

 a is the  slit width 

And θ is the angle made with the undeviated direction of light. 

The Central Maximum

The phenomena of light bending or diffraction may be seen in single-slit diffraction. Additionally, due to this phenomena, light from coherent sources interferes with itself, resulting in the creation of the diffraction pattern, a recognizable pattern on the screen.

For small θ,sin θ≈θ ⇒ λ = a sin θ≈aθ⇒ θ = y/D = λ/a⇒ y = λD/a⇒ Width of central maximum = 2λD/a⇒ Angular width of central maximum = 2θ = 2λ/a

###Young’s Single Slit Experiment

The double-slit experiment, carried out in 1801, proves that light is a wave. In this experiment, two tiny openings are illuminated by monochromatic light. After traveling through each slit, the waves superimpose to produce an alternative distribution of brightness and darkness on a distant screen. The brightness and size of each brilliant fringe are identical.

A similar pattern is shown on the screen when monochromatic light is fed through a single slit with a limited width in a single slit experiment. The breadth and intensity of the single-slit diffraction pattern decrease as we go away from the center maximum, in contrast to the double-slit diffraction pattern.

###Diffraction Maxima and MinimaMaxima: The intersection of two wavefronts, either at their crests position or troughs position.The maximum formula is provided by dsinθ=mλ

Minima: It is the intersection of two distinct wavefronts’ crests and troughs.The minima formula is provided by dsinθ= (m+1/2)λ

###Fringe Width

The distance between two succeeding bright or dark fringes is known as the fringe width. The band of alternately bright and dark material that results from interference is known as a fringe.

###SUMMARY 

• Diffraction is the curving of light waves around a tight turn of an obstacle or an opening. 
• In a single slit experiment, an alternating dark and bright pattern can be seen when light is imposed on a slit with a size corresponding to the wavelength of light. 
• The only differences between a single slit and a double-slit experiment are the diffraction patterns and the intensity graphs.

 

FAQs

Q. What is the condition for constructive interference?

For the constructive interference to take place, the phase difference between the two waves must be an even multiple of pie. 

Q. What is the condition for destructive interference?

For the destructive interference to take place, the phase difference between the two waves must be an odd multiple of pie.

Q. What is temporal coherence?

The average correlation between the value of a wave and itself delayed by any pair of times is known as temporal coherence.

We hope you enjoyed studying this lesson and learned something cool about Single Slit Diffraction! Join our Discord community to get any questions you may have answered and to engage with other students just like you! We promise, it makes studying much more fun!😎

]]>